A FULLY COUPLED FLUID-STRUCTURE INTERACTION ALGORITHM
Fluid-structure interactions, that is
interactions of some movable or deformable
structure with an internal or surrounding fluid
flow, are of great importance due to their many engineering
and biomedical applications. Accurate prediction of fluid-structure
interactions is crucial for many engineering
structures in order to avoid potential aeroelastic/hydroelastic
instability issues. Failing to consider these
effects can be catastrophic, especially in structures
comprising materials susceptible to fatigue. A new numerical
algorithm based on the ALE formulation has been developed
for a fully coupled solution of the large-scale FSI problems
where the fluid is modelled by the incompressible Navier-Stokes
equations and the structure is modeled by the St. Venant-Kirchhoff
model. The governing equations of the fluid domain are
discretized using an Arbitrary Lagrangian-Eulerian (ALE)
formulation based on the side-centered unstructured finite
volume method where the velocity vector components are defined
at the mid-point of each cell face while the pressure is defined
at the element centroid. The deformation of the solid domain is
governed by the constitutive laws for the nonlinear Saint
Venant-Kirchhoff material and the classical Galerkin finite
element is used to discretise the governing equations in
a Lagrangian frame. Newmark type generalized-alpha method
is employed to integrate in time the solid dynamic equilibrium
equation.
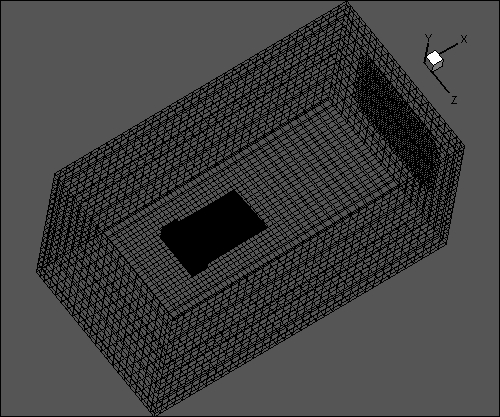
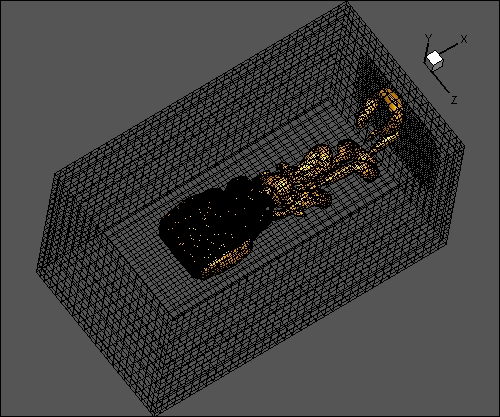
The computed vortex structure (Q-criteria) for a flat plate behind a rectangular cylinder at Re=648 (A. Eken).
The fluid-structure interaction witin a celabral artery with aneurysm (A. Eken).
The deformation of red blood cells (RBCs) in a narrow channel (A. Cetin).
The three-dimensional buckling of red blood cells (RBCs) in a narrow channel. Bending stiffness is significantly reduced (A. Cetin).
The three-link swimmer proposed by Eldredge, 2008 (E. Dilek).